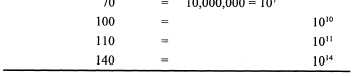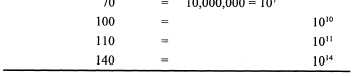In this system, the output of the signal is twice as
strong as the input to the system.
As you can see, this constant multiplication of the
ratios can be wearisome, and the products can be
extremely small or large. Therefore, the discovery
that adding the logarithms of the numbers would yield
the same result as this calculation led early scientists
to develop the unit of measure called the bel.
The bel, named in honor of Alexander Graham
Bell, expresses the logarithmic ratio between the input
and output of any given component, circuit, or
system. The bel maybe expressed in voltage, current,
sound levels, or power. The formula is as follows:
The gain of an amplifier can be expressed in bels
(N) by dividing the output (P1) by the input (P2) and
taking the base 10 logarithm (log 10) of the resulting
quotient. Thus, if an amplifier doubles the power, the
quotient will be 2. When you consult a logarithm
table, you will find that the base 10 logarithm of 2 is
0.3; so the power gain of the amplifier is 0.3 bel.
Experience has shown that the bel is a rather large
unit that is difficult to apply. A more practical, easier
unit to apply is the decibel (1/10 bel). Any figure
expressed in bels can be converted to decibels by
multiplying the value by 10. Thus the ratio of 0.3 bel
is equal to 3 decibels.
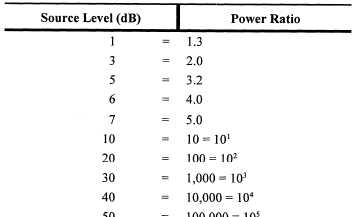
The reason the decibel system is used to express
signal strength is shown in table 1-2. For example,
saying that a reference signal has increased 50 dB is
much easier than saying that the output has increased
100,000 times.
The basis of the decibel measuring system is the
amount of increase or decrease from a reference level.
Whether the input power is increased from 1 watt to
1-4
100 watts or from 1,000 watts to 100,000 watts, the
amount of increase, or gain, is still 100 times or
20 dB. Examine table 1-2 again, taking particular
note of the power ratios for source levels 3 dB and 6
dB. As the table illustrates, an increase of 3 dB
represents a doubling of power. The reverse is also
true. If a signal decreases by 3 dB, half of the power
is lost. For example, a 1,000-watt signal decreased by
3 dB will equal 500 watts, while a 1,000-watt signal
increased by 3 dB will equal 2,000 watts.
Table 1-2.—Decibel Power Ratios
When you speak of the dB level of a signal, you
are actually speaking of the logarithmic comparison
between the input and output signals. The input
signal is normally used as the reference signal. In
some instances, a standard reference signal must be
used in place of the input signal. The most widely
used reference level is a 1-milliwatt signal (600-ohm
load). When the 1-milliwatt reference is used, the
standard decibel abbreviation of dB is changed to
dBm; dBms are used as an indication of power, while
dBs are used to indicate the ratio between the input
and output.
A signal level of +3 dBm is 3 dB above 1
milliwatt, and a signal level of –3 dBm is 3 dB below
1 milliwatt. Whether you are using dB or dBm, a plus
sign (+) or no sign indicates that the output level is